Practical compensation.
(Go back one page, or back to the
Introduction).
How do we properly set compensation? Proper compensation occurs
when, on average for a population, there is no contribution of
FITC fluorescence in the PE channel (and so on for other combinations
of fluorescent molecules). In this continuing example, this means
that a population of cells stained with fluorescein (but not PE)
should have the same median PE fluorescence as a population of
cells that is unstained for fluorescein. See Figure
4 (below). The compensation is increased until the center
of the positively stained population lines up with the center
of the negatively stained population. Because of the compounding
measurement error, this means that some of the cells will be above
a quadrant line set on the negatives! Indeed, note that if compensation
is set so that the positive population lines up underneath the
quadrant line, then the cells are actually over-compensated: on
average, most cells have too much signal subtracted, and the mean
PE fluorescence of this population is less than that of the negative:
less than autofluorescence. Obviously, this is incorrect.
In flow cytometric analyses on logarithmic axes, the median is
generally a much better estimate of central tendency than the
mean. The mean can be significantly skewed higher by a few outliers.
As well, we do not know the real fluorescence of an event appearing
on the lower axis (it could be any value below the minimum); thus
the mean will be artificially inflated by this as well. However,
neither process significantly affects the accuracy of the median.
Therefore, if possible, use the median fluorescence when doing
compensation: adjust compensation until the median of the positives
is equal to the median of the negatives (be sure to include all
cells in a population in the analysis gates).
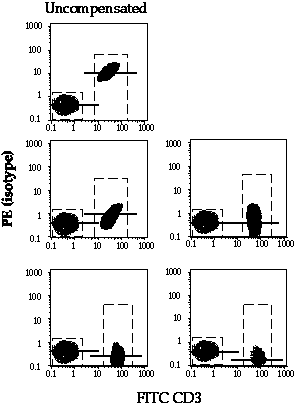
Figure 4: Practical
compensation

Legend: Cells were stained with FITC CD3 and PE Isotype
control. Correct compensation occurs when, for a population of
cells, there is no contribution of PE signal from the FITC signal.
In other words, proper compensation occurs when the FITC-positive
cells have the same mean (or median) PE fluorescence as the FITC-negative
cells (third panel in this series). The dashed boxes indicate
the analysis gates used when the mean (or median) fluorescences
were computed; the solid horizontal lines are drawn through the
median of each population.
By now, you can probably ascertain why none of the examples in
Figure 1B (in the quiz)
can be identified as properly compensated. In order to determine
the proper compensation, the medians of the positive and negative
population must be lined up. However, in Figure 1B, the negative
population is "smushed" against the bottom axis. Hence
the median fluorescence of this population cannot be determined
(it is less than 0.1, but we do not know how much less than 0.1).
See Figure 5 for a more detailed explanation.
Otherwise, go on.
Figure 5: Compensation
& sensitivity settings
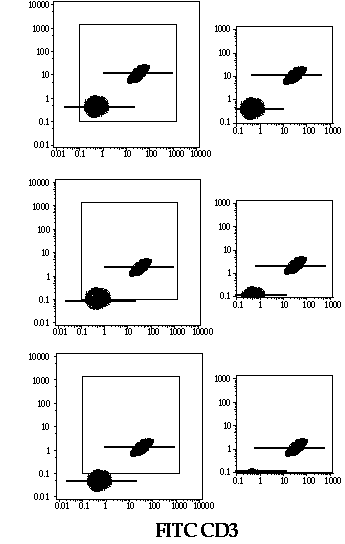
Legend:: In this Figure, there is no compensation
being done. The representation of cell fluorescences on a log
scale means that there is no position for true zero fluorescence
(because the log of zero is minus infinity). Our four-decade view
of the cells is simply a window onto the infinite range of possible
values. As the PMT voltage for PE is lowered, the two populations
of cells drop (and drop equal distances). On a six decade machine
(left), the cells would always be onscale, and the centers of
the populations could be aligned at any of these voltages. However,
on a four decade machine, cells which fall outside of the window
appear on the axis. This means that the true fluorescence of these
cells is no longer known--it is impossible to know if the cell
was at 0.02, 0.05, or 0.1. Therefore, the center of this population
that is pinned to the axis becomes the axis value itself (0.1).
The effect on compensation is substantial. The two cell populations
are separated by a factor of about 100 in the FITC channel, and
about 20 in the PE channel: this means that proper compensation
will be 20%. But in the bottom right example, the separation between
the mean of the negatives and the positives is only about 10-fold:
leading to a compensation setting of 10%, which is too low.
Go on.